Let X Be a Poisson Random Variable With Parameter

PX is even 121 e2λ by using the result of Theoretical Exercise 15 and the relationship between Poisson and binomial random variables. Eλ X k0 rλk k.

Pin By Swapnil Parkhe On Clv Poisson Distribution Exponential Journal

Wedraw a ball out of the urn 10 times taking care to replace the ball and shake up the urn between draws.

. Let X denote an exponential random variable with unknown parameter λ0. To get that last line find the absolute value of the Jacobian determinant. λ λ 2 10 λ 10.

Fxdfrace-lambda lambdaxx for x0 1 2 ldots and lambda0 where lambda will be shown later to be both the mean and the variance of X. Let X and Y be. Sums of independent Poisson random variables are Poisson random variables.

Gr eλerλ eλr1 3. P X x e λ λ xx where λ is a parameter. SOLVEDProblem Let X and Y denote two independent Poisson random variables with parameter Ax and Ay.

Let Xbe a Poisson random variable with parameter. 3 points Compute EX. This problem has been solved.

PX 1 X 2 z X1 i0 PX 1 X 2 zX 2 i X1 i0 PX 1 i zX 2 i Xz i0 PX 1 z iX 2 i z i0 PX 1 z iPX 2 i. Let X be a Poisson random variable with parameter lambda. B Verify the formula in part a directly by making use of the expansion of eλ.

An urn contains 9 balls 4 of which are red and 5 of which are blue. B Simplify the above expression X is even by utilizing the following. If true determine its parameter.

A random variable X has a Poisson distribution with parameter λ such that P X 1 02 P X 2. Recall the definition of the indicator function here is IX51ifX50ifX5. Let X be a Poisson random variable with parameter.

Poisson random variables with parameter λ0 and denote by Xn their empirical average Xn1ni1nXi. 02e λ λ 22. Poisson distribution Let X be a Poisson random variable with parameter λ.

U x y v x x y x u v y u 1 v d x d y u d u d v. Answer the following True False problems You need justify your answers_ 1. Find step-by-step Probability solutions and your answer to the following textbook question.

Given that P x 1 02 P X 2 e λ λ 11. Eλ λeλ X x0 λx1 x1. Let X1Xn be iid.

λeλeλ λ Remarks. B Calculate the probability that X takes even integers. Lets find stationary points.

Use tables for means of commonly used distribution. Now substitute λ 10 in the formula we get. Verify the formula in part a directly by making use of the expansion of e-λ e λ.

Solution for O Let X be a Poisson random variable with parameter 0 then E X² 1. P Zz PZ z Xz j0 PX j Y z j so X Y z Xz j0 PX jPY z j since Xand Y are independent Xz j0 e 1 j. We think of Y as a.

Scholz Poisson-Binomial Approximation Theorem 1. De ne 1 2 and Z X Y. A Calculate EX EX X 1 and EX X 1 X.

Let X 1 and X 2 be independent Poisson random variables with respective parameters 1 0 and 2 0. Let X be a Poisson random variable with parameter λ 2 and let Y be a geometric random variable with parameter p -Suppose that X and Y are independent and let Z -X Y. X k1 λeλ λk1 k 1.

ErX X k0 rkeλ λk k. Let X be a Poisson random variable of parameter λ. Let X be a Poisson random variable with parameter λ.

So by definition we have the expected value of X is equal for the is equal to the summation for all x of x times The probability at X This is the. A What is the probability that X is even. Then S X 1 X 2 is a Poisson random variable with parameter 1 2.

The solution is λ k lambda k λ k. K 1 k1 1 if k 1 PX i increases monotonically and then decreases monotonically as i. P X 0.

3 points Compute gr E rX for any real number r 0. Find P X 0. λeλ X k0 λk k.

Show that PXi increases monotonically and then decreases monotonically as i increases reaching its maximum when i is the largest integer not exceeding lambda. Let X be a Poisson random variable with parameter λ. The Poisson Random Variable A random variable X taking on the values 0 1 2 is said to be a Poisson random variable with parameter λ if for some λ 0.

P X k lo g λ k e λ k lo g λ λ. ZX2 is a Poisson random variable. Determine its parameter.

E X X x0 x λx x. By using the result of Theoretical Exercise 415 and the relationship between Poisson and binomial random variables. Show that PXk1 PXk 1 PX k 1 PX k e k1 k1.

For the Poisson distribution the probability function is defined as. Show that PX i increases monotonically and then decreases monotonically as iincreases reaching its maximum when iis the largest integer not exceeding Solution. Since λ k lambda k λ k is the only stationary point we have that for that λ lambda λ density function P X k P Xk P X k maximises.

EX X k0 kPX k X k0 keλ λk k. For most distributions some advanced knowledge of calculus is required to find the mean. If X is a Poisson random variable then the probability mass function is.

Claim that Zis a Poisson random variable with parameter. For each of the following statements state whether it is true meaning always true or false meaning not always true. One way to look at this is a change of variables.

Gxpxtan10 1 6 tan11 5 6 0 π 4 5 6 5π 24. Z XY is a Poisson random variable_ If true. There should also be a nice geometric argument involving infinitely small increments.

Find two sequences ann1 and bnn1 such that an Xnbn converges in distribution to a standard Gaussian random variable ZN 01. Let X be a Poisson random variable with parameter 0. In this exercise we show that if X is a Poisson random variable with parameter mu its expected value is equal to mu and were asked to do this by starting with the definition for expected value.

Let YIX5 the indicator that X is larger than 5. A family has children with probability qp n n 1 where α 1 - pp. Let Xand Y be independent Poisson random variables with parameters 1 and 2 respectively.

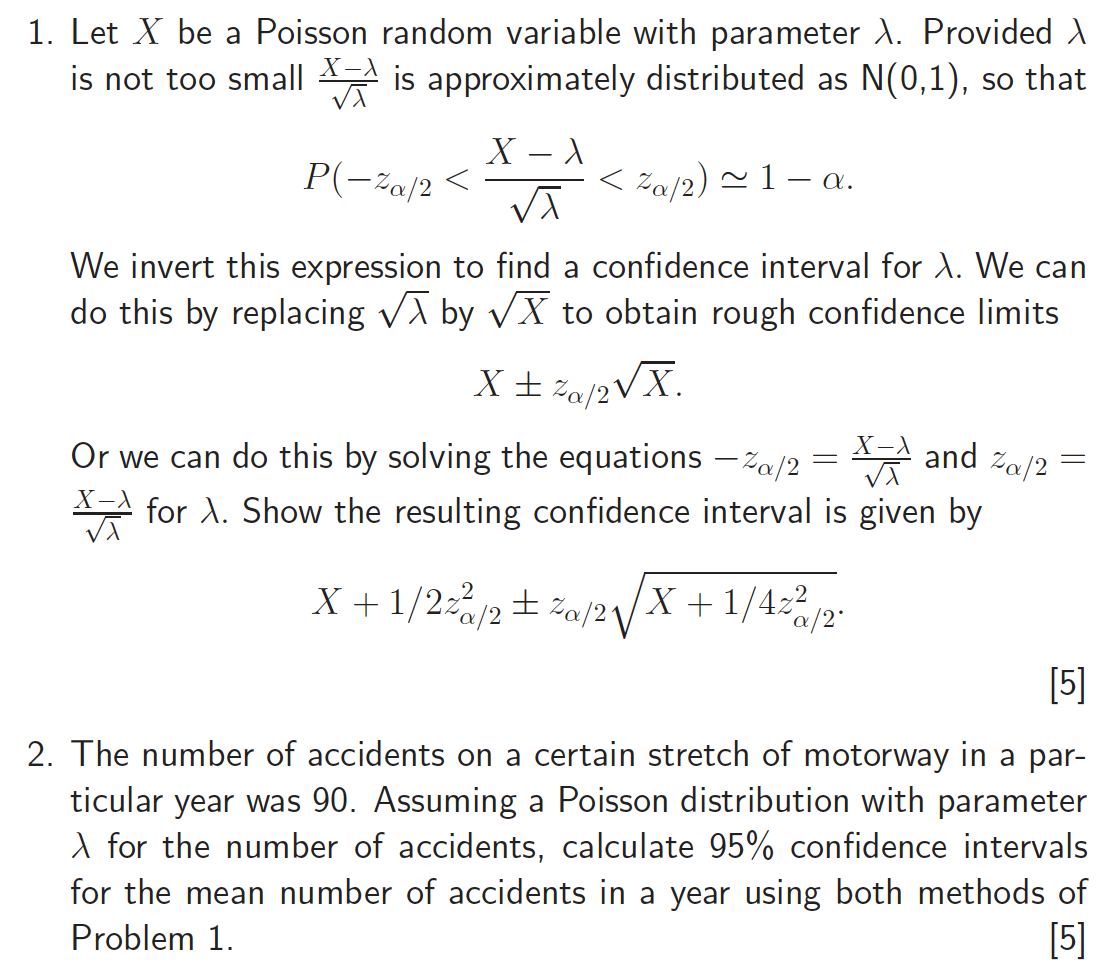
Solved 1 Let X Be A Poisson Random Variable With Parameter Chegg Com

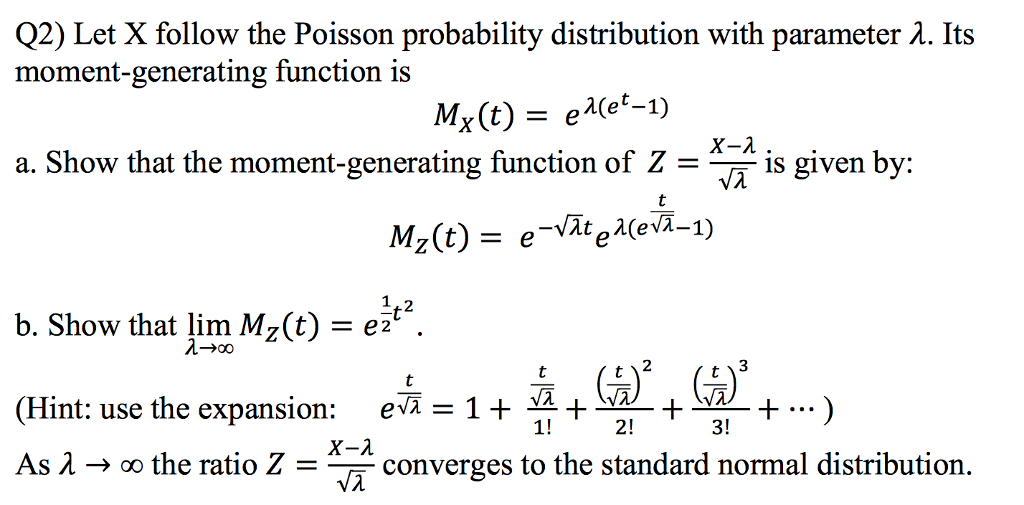
Solved Let X Follow The Poisson Probability Distribution Chegg Com

Solved Problem 4 Let X Be A Poisson Random Variable With Chegg Com